is one of the broad parts of mathematics, together with number theory, geometry and analysis. In its most general form, algebra is the study of mathematical symbols and the rules for manipulating these symbols; it is a unifying thread of almost all of mathematics.
Algebra began with computations similar to those of arithmetic, with letters standing for numbers.[8] This allowed proofs of properties that are true no matter which numbers are involved. For example, in the quadratic equation:
can be any numbers whatsoever (except that cannot be ), and the quadratic formula can be used to quickly and easily find the value of the unknown quantity .
As it developed, algebra was extended to other non-numerical objects, such as vectors, matrices, and polynomial. Then the structural properties of these non-numerical objects were abstracted to define algebraic structures such as groups, rings, and fields.
Instead of saying "obviously x=2", use this neat step-by-step approach:
- Work out what to remove to get "x = ..."
- Remove it by doing the opposite
- Do that to both sides
And what is the opposite of multiplying? Dividing!
Have a look at this example:
| We want to remove the "4" | To remove it, do the opposite, in this case divide by 4: | Do it to both sides: | Which is ... | Solved! |
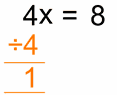 | 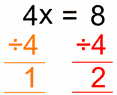 |

 can be any numbers whatsoever (except that
can be any numbers whatsoever (except that  cannot be
cannot be  ), and the quadratic formula can be used to quickly and easily find the value of the unknown quantity
), and the quadratic formula can be used to quickly and easily find the value of the unknown quantity  .
.
No comments:
Post a Comment